Mathematical Models of Stratfordian Persistence

by Dr. Charles Berney

The year 1593 saw the publication of the first work attributed to “William Shake-speare, ” the narrative poem Venus and Adonis. Subsequent years saw other publications with this attribution: another poem, a number of plays, a collection of sonnets, and finally, in 1623, a large volume entitled Mr. William Shakespeares Comedies, Histories, & Tragedies appeared, containing thirty-six plays. This collection, usually referred to as the First Folio, contained introductory material eulogizing the deceased author, but provided remarkably little biographical information. Ben Jonson addresses the author as “Sweet Swan of Avon!” and Leonard Digges refers to “thy Stratford Moniment.” These references have been interpreted to mean that the author was William Shaksper, a native of the village of Stratford-on-Avon. This interpretation, bolstered by further events, such as the appearance of Nicholas Rowe’s biography (1709) and David Garrick’s Stratford Festival (1769), became the orthodox belief.

The first major public challenge to belief in the Stratfordian Shakespeare occurred in 1856 with the publication of Delia Bacon’s book, The Philosophy of the Plays of Shakespeare Unfolded. Bacon argued that the plays had been written by a group of Elizabethan courtiers including Francis Bacon (no relation), Walter Raleigh, and Edmund Spenser, an assertion that in public debate soon took on the simpler form, “Bacon wrote Shakespeare.” This debate stirred up interest in the authorship question, and several other candidates were proposed, e.g. Christopher Marlowe, the Earl of Derby, and the Earl of Rutland. The paucity of evidence for the Stratfordian attribution was authoritatively summarized in George Greenwood’s book The Shakespeare Problem Restated (1908), a work which inspired Mark Twain’s essay “Is Shakespeare Dead?” (1911). Both Greenwood and Twain are careful to point out that they are not claiming that Bacon was the author; rather that it was impossible that it could be the Stratford man.

The modern phase of the authorship question began in 1920 with the publication of a book by John Thomas Looney, “Shakespeare” Identified. Looney was an English schoolmaster who had taught Shakespeare’s plays for many years and had grown skeptical of the traditional attribution. He embarked on a systematic search for the identity of the true author, armed with a set of characteristics (e.g., “A member of the higher aristocracy … Loose and improvident in money matters … “) gleaned from Looney’s study of the plays.

Looney’s special insight was that an author writing Shakespeare’s works pseudonymously would not have had time for a second career; thus he looked for someone who was relatively unknown, rather than a famous figure like Bacon or Raleigh. He started out by scanning an anthology of Elizabethan poetry, looking for contributors who wrote in the meter used in Venus and Adonis. He found a candidate in Edward de Vere, Seventeenth Earl of Oxford, and further research showed that the set of required characteristics Looney had derived fit Oxford like a glove. He summarized his research in his book, which is still a thrilling account of a major discovery, beautifully written, cogently argued, and utterly compelling.

The subject I will examine here is the response to this book of the academic community, loosely defined as the ensemble of all professors teaching English literature in four-year degree-granting colleges, or in associated graduate studies. In accordance with current usage, those who are firmly committed to the view that Shaksper of Stratford was the author of Shakespeare’s works will be termed Stratfordians, while those convinced by Looney’s arguments will be designated Oxfordians. A further category comprises those who believe that Oxford wrote the works, but find it politically disadvantageous to admit it, the cryptoOxfordians. (As the term implies, the identities of crypto-Oxfordians are hidden, but I have my own favorite candidates, including Helen Vendler, author of The Art of Shakespeare’s Sonnets, whose insistence that Shakespeare writes with the voice of a “fictive speaker” forestalls discussion of any possible autobiographical elements, and Louis Marder, who is surely aware that printed etchings reverse the original image.) To simplify the discussion, we will assume that all academics belong to one of these categories, so

P = P_St + P_Ox + P_COx

where P is the population of academics, and the subscripted quantities correspond to the categories defined above. For further simplification, the variable we will be dealing with is

p = P_St/P

the fraction of the population composed of committed Stratfordians. Specifically, we will examine possible models of the time evolution of the Stratfordian population, p(t).

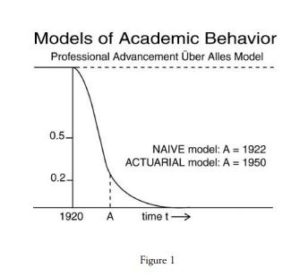Figure 1 illustrates a possible model for academic behavior: we assume that prior to the appearance of Looney’s book, the population is entirely Stratfordian (p = 1). Then in 1920, professors start reading about the Oxfordian hypothesis, and realize that it solves many nagging questions raised by the Stratfordian attribution, and that in addition, it makes possible a depth and coherence of understanding of the works that was previously unattainable.
The Stratfordian population then drops rapidly, so that after about a year, has declined to about 0.20. This model obviously does not account for the observed facts, so it must be termed the Naive Model of academic behavior. A more realistic model (the Actuarial Model) might be devised from the following scenario: we assume that the senior faculty (say 50 years old in 1920) guard the gates of orthodoxy while 20-year-old grad students read Looney on the sly. In about 30 years (-1950) the former grad students are the senior faculty, and the Oxfordian attribution has become orthodox. Again, this model fails to account for the fact that English departments are still (apparently) overwhelmingly Stratfordian.

A much more successful scheme is the Professional Advancement Über Alles Model, which assumes that the promotion of a young Shakespeare scholar depends on his or her writing a book that is well received by the Old Guard. Since the Old Guard is Stratfordian, the successful book will be written from a Stratfordian viewpoint, even if the authorship question is not explicitly discussed. Over time, as the successful young author him/herself becomes a member of the Old Guard, it will of course be impossible to disown the earlier work, so the Stratfordian orthodoxy is stable and self-perpetuating for all time, as indicated by the horizontal line p(t) = 1.0 at the top of Fig. 1.

While the above model is, sadly, the most accurate one considered so far, there are indications that it is unduly pessimistic: at the time of writing I am aware of at least two English departments (Concordia University in Portland, Oregon, and the US Air Force Academy in Colorado Springs) headed by Oxfordian scholars, and the de Vere Studies Conference, organized at Concordia University by Dr. Daniel Wright, is about to have its fourth annual meeting. So p(t) must be diminishing, at however glacial a pace. We therefore propose a Modified Professional Advancement Model. Again, we assume that the academic population consists of Stratfordians, Oxfordians, and crypto-Oxfordians, that p(t) is the fraction of committed Stratfordians, and that the time variable t is the number of years after 1920. We assume that p(O) = 1.0 (that is, in 1920, all academics were Stratfordians). We then assume there is a gradual decay in p(t) (that is, some academics become Oxfordians or crypto-Oxfordians) until p(t) = 0.5. That represents the point at which Oxfordians and crypto-Oxfordians are as numerous as Stratfordians, and there is thus little reason for hiding one’s Oxfordian proclivities. It is therefore a critical point initiating a change of phase in the system (analogous to a warming ice crystal when temperature T(t) reaches 0° Centigrade).

The Modified Professional Advancement Model is capable of yielding quantitative results if a suitable function is chosen to describe the decay of p(t). The function that comes immediately to mind is the exponential function,

where the notation “exp” indicates that the constant e (2.718 … ) is to be raised to the power represented by the quantity in parenthesis (this function is widely used to represent a number of physical processes, including radioactive decay). As noted above, t is the time in years since 1920; r

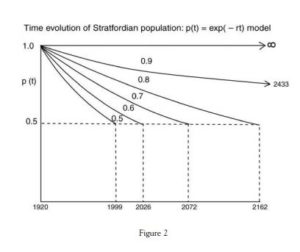
is a rate constant which we will call the “academic rationality index,” a quantity which can range from 1 to 0. If r = 1 we get complete conversion to the Oxfordian attribution within a few months, thus reproducing the Naive Model illustrated in Fig. 1. If r = 0 the rate of Stratfordian decay is zero, reproducing the simple Professional Advancement Model (dashed horizontal line in Fig. 1). Since the former model is too optimistic and the latter is too pessimistic, we know that the value of r which best fits the actual situation is less than 1 and greater than 0. If we knew the value of p(t) for any given time, we could solve for r and use that value to predict the year in which p(t) would reach the critical value of 0.5. Unfortunately, estimating p(t) requires knowing the population of crypto-Oxfordians, P_cOx and no experimental method for determining this number has yet been devised (by the definition of a crypto-Oxfordian, questions about authorial orientation will not be answered candidly). The best we can do is assume a series of values of p for the year 1999, solve for r, then calculate the critical year in which p(t) 0.5. The results for a series of these calculations are shown in Table 1 and plotted in Figure 2. Table 1, for example, shows that if p(t) in 1999 were 0.7, it would reach 0.5 by the year 2072, 73 years from 1999, a discouraging prediction, since the author does not expect to be around in 2072.

As mentioned above, the exponential function is used for modeling the process of radioactive decay. One of the physical features of this process is that the radioactive nuclei do not interact with each other; the probability that any given nucleus will decay in any given period of time is entirely independent of what the other nuclei are doing, and from this standpoint, a sample of radioactive material can be regarded as a collection of noninteracting particles. It may be that the simple exponential function is inadequate to describe English departments, where there is at least the possibility that members might interact.

This possibility could be accounted for mathematically by increasing the time dependence of the describe the decay. function used to This can be done most simply by raising t in the exponent to a higher power:

The results of using this function to solve for a series of values of r using assumed values for p are

shown in Table 2 and plotted in Figure 3. Examination of Fig. 3 shows us what we should have recognized immediately, that exp(-re) is a Gaussian function, widely used in population studies. Table 2 shows us that the Gaussian function is more optimistic than the simple exponential: if p(t) is 0.7 in 1999, it is predicted to reach the critical value of 0.5 in 2029, when some of us may still be around.

Switching from exp(-rt) to exp(-re) changes the dimension of the academic rationality index r from (years)-1 to (years)-2. Note the extraordinarily small values of r in Table 2: if 0586 (equivalent to 58.6 microberneys, where a berney is taken as the standard unit of rationality).

In one sense this has been an empty exercise: we don’t know the current value of p, we’re not sure that the dynamics are Gaussian, we’re not even sure that 0.5 is the critical value of p (perhaps the power of senior faculty is such that p must fall to 0.4 or O.3 before a paradigm shift occurs). However, for some people, it is satisfying to have constructed a mathematical model, even if it is a fuzzy one; the parameters have been defined, even if their exact values are not known. Certainly efforts could be made to get a firmer estimate of p; perhaps a crypto-Oxfordian hotline could be established to gather anecdotal evidence. It may be that one value of this study is to clarify the
time perspective. 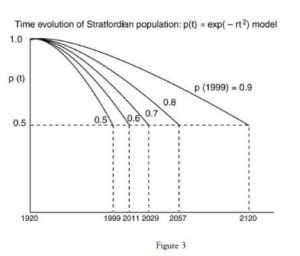I first read Charlton Ogburn’s great book, The Mysterious William Shakespeare, in 1986, and it seemed to me that the case he made for Oxford’s authorship was obvious and irrefutable. If I had been told then that thirteen years later the orthodox view would still be firmly established, I would have been astonished. The Gaussian model tells us that even if the current academic population is 30 percent Oxfordian and crypto-Oxfordian it will be another 30 years before that view prevails. We should be prepared for a long haul.